Quantum collision models
Giorno 27 gennaio 2020, con inizio alle ore 9:00, presso l'Aula D del DFA, su invito dei Professori Giuseppe Falci e Alessandro Ridolfo, il Dr Francesco Ciccarello (NEST, Istituto Nanoscienze-CNR & University of Palermo, Italy) terrà un seminario/lezione dal titolo: Quantum collision models: a tutorial.
Tutti gli interessati sono invitati a partecipare.
Abstract.
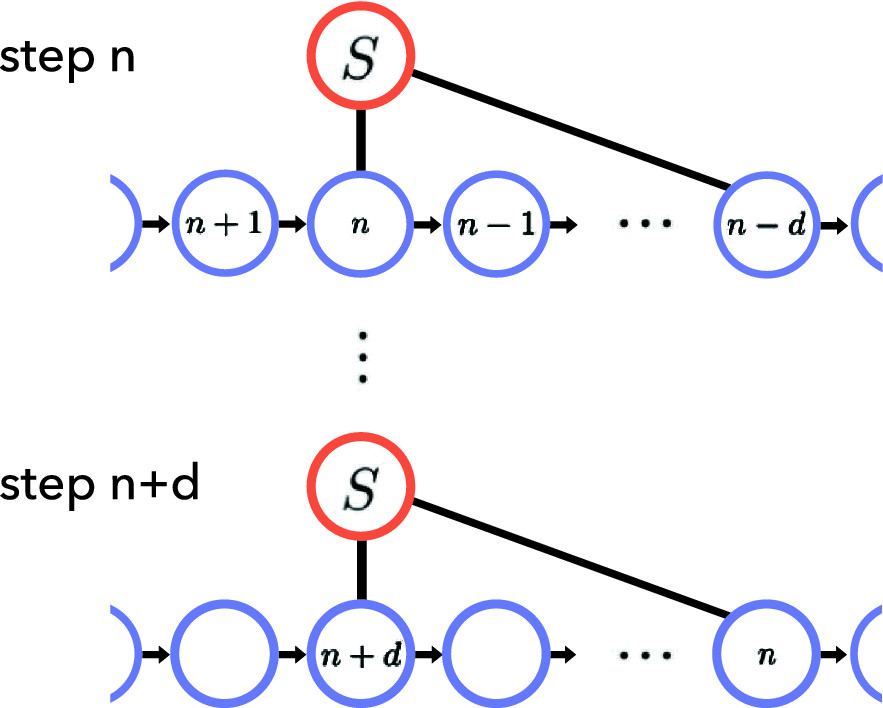
A collision model (or repeated-interactions model or conveyor-belt model) is a simple theoretical framework in which a system S undergoes successive interactions (“collisions”) with the subunits of a large environment [1-4]. Currently, quantum collision models are being used more and more in research areas such as non-Markovian quantum dynamics [5], quantum thermodynamics (where they became a standard tool by now) [6], quantum optics [7] and, in some respects, even quantum gravity [8].
This tutorial aims at introducing some basic concepts of Markovian quantum collision models. Given the remarkable simplicity of collision models and their intimate connection with a number of central concepts/tools in open quantum systems theory - such as quantum maps, the Stinespring dilation theorem, the Lindblad master equation and quantum trajectories - the tutorial can also be seen as a pedagogical, short introduction to open quantum systems theory itself.
The standard model of quantum optics treating the field as a white-noise bosonic bath is an important microscopic scenario which can be exactly mapped into a collision model. We will show this in some detail and use the same framework for the classroom demonstrations (second seminar). These will illustrate how spontaneous/stimulated decay and optical Bloch equations can be derived ab initio from a fully quantum atom-field model through a collision-model approach.
Required background:
- elementary quantum mechanics;
- a minimum acquaintance with atom-field interaction when the field is quantized;
- a minimum familiarity with the density-matrix language would help, although we will briefly review it in the beginning.
References:
- J. Rau, Phys. Rev. 129, 1880 (1963).
- C. M. Caves and J. G. Milburn, Phys. Rev. A 36, 5543 (1987).
- T. A. Brun, Am. J. Phys. 70, 719 (2002).
- V. Scarani M. Ziman, P. Stelmachovic, N. Gisin, and V. Buzek, Phys. Rev. Lett. 88, 097905 (2002).
- F. Ciccarello, G. M. Palma, and V. Giovannetti, Phys. Rev. A 87, 040103(R) (2013).
- P. Strasberg, G. Schaller, T. Brandes, and M. Esposito, Phys. Rev. X 7, 021003 (2017).
- A. Grimsmo, Phys. Rev. Lett. 115, 060402 (2015); H. Pichler and P. Zoller, Phys. Rev. Lett 116,093601(2016); F. Ciccarello, Quantum Meas. Quantum Metrol. 4,53 (2017); J. A. Gross, C. M. Caves, G. J. Milburn, and J. Combes, Quantum Sci. Technol. 3, 024005 (2018).
- N. Altamirano, P. Corona-Ugalde, R. Mann, and M. A. Zych, New J. Phys. 19, 013035 (2017).